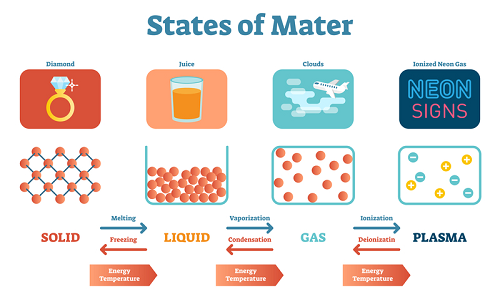
根据分子之间的吸引力，物质分为五类。它们是固体、液体、气体、等离子体和玻色-爱因斯坦。固体是指分子之间的距离很小并且彼此紧密结合的物质。液体是分子距离很小的物质，它们的结合不如固体紧密。等离子体态分子具有高动能。

Assumptions of Kinetic Theory of Gases

气体的动力学理论显示了气体的热力学行为。他们有一些基本假设，

气体中有许多被称为粒子的微小粒子。

它们相互之间以及与容器的壁发生弹性碰撞。

粒子彼此相同，可以在所有可能的方向上移动。

与碰撞之间的时间相比，碰撞的时间非常小。

与气体的总体积相比，分子的体积要小得多。

分子不会对另一个施加任何力，除非在碰撞过程中。

与分子的大小相比，分子之间的分离更大。

所有的分子都服从运动定律。

What do you mean by Kinetic Interpretation of Temperature ?

让我们考虑体积为V的充气容器。让分子以所有可能的速度运动。然后，由气体分子施加在壁上的压力由，

$mathrm{P=frac{1}{3}frac{mnc^2}{v}}$

其中m表示一个分子的质量。n表示分子的数量$mathrm｛C^2｝$表示分子的均方根速度。V表示气体的体积。

1摩尔的气体含有N个分子。

$mathrm{PV=frac{1}{3}mNC^2}$

根据气体定律PV=RT

$mathrm{RT=frac{1}{3}mNC^2}$

$mathrm{frac{RT}{N}=frac{1}{3}mC^2}$

将右边乘以2，我们得到，

$mathrm{frac{RT}{N}=frac{2}{3} imes frac{1}{2} mC^2}$

分子的动能为$mathrm｛K.E＝frac｛1｝｛2｝mC^2｝$

$mathrm{frac{RT}{N}=frac{2}{3} imes K.E}$

$mathrm{K.E=frac{3}{2} imes frac{RT}{N}}$

$mathrm{K.E=frac{3}{2} KT}$

其中$mathrm{K=frac{R}{N}}$表示玻尔兹曼常数。

Relation between Kinetic Energy and Temperature

对身体所做的工作就是它的能量。由于运动而拥有能量的物体称为动能。它可以从一个物体转化为另一个物体，也可以转化为其他形式的能量。这个能量取决于它的质量和速度。动能的S。I单位是焦耳。因此，物体的动能由，

$mathrm{K.E=frac{1}{2} mc^2}$

分子的自由度因能量均分定律而不同；系统的总能量被平均分配给分子的每个自由度。平均分配的能量是1/2 kT，这就是能量均分定律。因此，气体的温度与分子的动能直接相关。

Gas laws

为了了解气体的行为，人们提出了许多法律。气体定律给出了压力、温度和体积之间的关系。它们是查理定律、盖伊·卢萨克定律、阿伏伽德罗定律、波义耳定律和格拉姆定律。

博伊尔定律：博伊尔定律清楚地表明，气体的压力与气体的体积是有争议的。（即）当压力增加时，体积将减小。只要温度恒定，这种情况就会一直保持下去。数学表示给出，

$mathrm{P varpropto frac{1}{v}}$

$mathrm{PV = k:(constant)}$

查理定律：当温度升高时，气体会膨胀。这可以用查理定律来解释。结果表明，当压力保持不变时，气体的体积直接随温度的变化而变化。

$mathrm{V varpropto T}$

$mathrm{frac{v}{T} = k(constant)}$

Gay-Lussac定律：当气体体积保持不变时，气体的压力直接随着温度的变化而变化。这就是众所周知的盖伊·卢萨克定律。

$mathrm{P varpropto T}$

$mathrm{frac{P}{T} = k(constant)}$

阿伏伽德罗定律：阿伏伽dro定律说，在相同体积的气体中，当相同的温度和压力相同时，分子的数量将相同。

$mathrm{V varpropto n}$

$mathrm{frac{v}{n} = k}$

格雷厄姆定律：根据他的原理，较轻的分子比较重的分子移动得更快。即，在恒定的压力和温度下，低分子质量的分子的渗出速度比高分子质量的分子更快。分子随时间的扩散是其质量密度平方根的倒数。

$mathrm{r varpropto frac{1}{sqrt{P}}}$

Conclusion

在本教程中，详细讨论了气体动力学理论的假设和温度的动力学解释。还讨论了动能与温度和气体定律之间的关系。

FAQs

问题1.解释自由度

答：分子提及其位置所需的数字坐标称为自由度。一般来说，单原子分子有三个自由度。具有一个以上原子的分子包括另外三个自由度，如平移运动、旋转运动和振动运动。根据这个自由度，具有一个以上原子的分子将发生变化。

Q2.状态是指气体中的自由路径

答：在气体中，分子相互碰撞，也与容器壁碰撞。分子在任意两次连续碰撞之间行进的距离的平均值被称为平均自由程。用λ表示。

Q3.影响气体行为的因素是什么

答：影响气体行为的因素有温度（T）、压力（P）、体积和数量。如果气体的温度升高，气体就会膨胀，从而体积增加。类似地，随着温度的降低，体积也由于气体分子的压缩而减小。温度的升高导致气体膨胀，因此其压力增加，反之亦然。要将气体转化为固体或液体，温度必须非常低。数量和压力成正比。由于压力与体积和数量成正比，因此通过使体积和数量变高来增加压力。

Q4.列出气体定律的一些应用

答：如果环境条件发生变化，那么气体分子的行为就会偏离它们的行为。气体定律可以很好地研究这些变化。用于吸入液体的注射器是根据波义耳定律原理设计的。蛋糕和面包在加热时的膨胀符合查理定律。它也被用于天气预报系统。它们也用于热力学和流体动力学。

问题5.气体是如何被压缩的

答：气体是物质的一种状态，在这种状态下分子是松散结合的。所以分子之间有很多间隙。当对气体施加压力时，间隙会减小，颗粒会越来越近。从而体积减小并且气体被压缩。